Angular velocity

src: i.ytimg.com

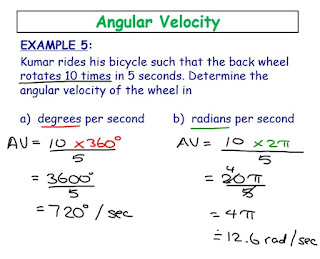
In physics, the angular velocity of the particle is the rate at which it rotates around the selected center point: that is, the time level of the angular change of displacement relative to the origin. It is measured in angles per unit of time, radians per second in SI units, and is usually represented by the omega symbols (? , sometimes ? ). By convention, the positive angular velocity represents a counterclockwise rotation, while negative clockwise.

For example, geostationary satellites complete one orbit per day above the equator, or 360 degrees per 24 hours, and have angular velocity ? = 360/24 = 15 degrees per hour, or 2?/24? 0.26 radians per hour. If the angle is measured in radians, linear velocity is the radius times the angular velocity, ${\ displaystyle v = r \ omega}$ . With an orbital radius of 42,000 km from the center of the earth, the satellite velocity through space is v = 42,000 ÃÆ'â € "0.26? 11,000 km/h. The angular velocity is positive because the satellite moves eastward with the Earth's rotation (counterclockwise from above the north pole.)

In three dimensions, the angular velocity is a vector, by the magnitude of measuring the rotation rate, and its direction points along the axis of rotation (perpendicular to the radius and vector velocity). The upward or downward orientation of the conventional angular velocity is determined by the right-hand rule.

Video Angular velocity

Particle angle velocity

Particles in two dimensions

Dalam kasus gerakan melingkar paling sederhana pada radius r , dengan posisi yang diberikan oleh perpindahan sudut ${\ displaystyle \ phi (t)}  ÂÂ$ dari sumbu x, kecepatan sudut adalah laju perubahan sudut terhadap waktu: ${\ displaystyle \ omega = {\ tfrac {d \ phi} {dt}}}  ÂÂ$ . Jika ${\ displaystyle \ phi}  ÂÂ$ diukur dalam radian, jarak dari sumbu x di sekitar lingkaran ke partikel adalah ${\ displaystyle \ ell = r \ phi}  ÂÂ$ , dan kecepatan linier adalah ${\ displaystyle v (t) = {\ tfrac {d \ ell} {dt}} = r \ omega (t)}  ÂÂ$ , sehingga ${\ displaystyle \ omega = {\ tfrac {v} {r}}}  ÂÂ$ .

In the general case of particles moving in the plane, the angular velocity is measured relative to the selected center point, which is called origin. The diagram shows the radius vector r from the O to the particle P , with its polar coordinates ${\ displaystyle (r, \ phi)}$ . (All variables are time function t .) The particle has a linear velocity separation as the ${\ displaystyle {\ mathbf {v}} = {\ mathbf {v}} _ {\ |} {\ mathbf {v}} _ {\ perp }}$ , with the radial component ${\ displaystyle {\ mathbf {v}} _ {\ |}}$ ${\ displaystyle {\ mathbf {v}} _ {\ perp}}$ perpendicular to the radius. When there is no radial component, the particle moves around the origin of the circle; but when there is no cross-radial component, it moves in a straight line of origin. Because the radial motion left the angle unchanged, only the cross-radial component of linear velocity contributes to angular velocity.

Kecepatan sudut ? adalah laju perubahan sudut terhadap waktu, yang dapat dihitung dari kecepatan lintas-radial sebagai:

${\ displaystyle \ omega = {\ frac {d \ phi} {dt}} = {\ frac {v _ {\ perp}} {r}}.}  ÂÂ$

Di sini, kecepatan lintas-radial ${\ displaystyle v _ {\ perp}}  ÂÂ$ adalah ukuran bertanda ${\ displaystyle {\ mathbf {v}} _ {\ perp}}  ÂÂ$ , positif untuk gerakan berlawanan arah jarum jam, negatif untuk searah jarum jam. Mengambil koordinat polar untuk kecepatan linier v memberikan besaran v (kecepatan linier) dan sudut ? relatif terhadap vektor radius; dalam istilah ini, ${\ displaystyle v _ {\ perp} = v \ sin (\ theta)}  ÂÂ$ , jadi itu

${\ displaystyle \ omega = {\ frac {v \ sin (\ theta)} {r}}.}  ÂÂ$

In two dimensions, the angular velocity is a number with a plus or minus sign indicating orientation, but not pointing to a direction. This mark is conventionally considered positive if the radius vector changes counterclockwise, and negative if clockwise. Angular velocity can be called pseudoscalar, numerical quantity that alters sign under partial inversion, such as inverting one axis or replacing two axes.

Particles in three dimensions

In a three-dimensional space, we again have a position vector r of the moving particle, its radius vector from the origin. The angular velocity is a vector (or pseudovector) whose magnitude measures the rate at which the radius sweeps an angle, and whose direction indicates the rotation axis. The upward or downward direction is given by the right hand rule.

Let the axial vector ${\ displaystyle {\ mathbf {u}}}$ into a one-to-one vector perpendicular to the rotation field spaced by the radius and vector velocity, so the rotation direction is counter-clockwise looking from above span> ${\ displaystyle {\ mathbf {u}}}$ . Retrieve polar coordinates ${\ displaystyle (r, \ phi)}$

                        r                                                u                   ,           {\ displaystyle {\ boldsymbol {\ omega}} = \ omega {\ mathbf {u}} = {\ tfrac {d \ phi} {dt}} {\ mathbf {u}} = {\ frac {v \ sin (\ theta)} {r}} {\ mathbf {u}},}  Â

di mana ? adalah sudut antara r dan v . Dalam hal produk silang, ini adalah:

${\ displaystyle {\ boldsymbol {\ omega}} = {\ frac {{mathbf {r}} \ kali {\ mathbf {v}}} {r ^ {2 }}}.}  ÂÂ$

Dari sini, seseorang dapat memulihkan kecepatan tangensial sebagai:

${\ displaystyle {\ mathbf {v}} _ {\ perp} = {\ boldsymbol {\ omega}} \ kali {\ mathbf {r}}}  ÂÂ$

Penambahan vektor kecepatan sudut

Jika sebuah titik berputar dengan kecepatan sudut ${\ displaystyle \ omega _ {1}}  ÂÂ$ dalam bingkai koordinat ${\ displaystyle F_ {1}}  ÂÂ$ yang dengan sendirinya berputar dengan kecepatan sudut ${\ displaystyle \ omega _ {2}}  ÂÂ$ berkenaan dengan bingkai eksternal ${\ displaystyle F_ {2}}  ÂÂ$ , kita dapat mendefinisikan ${\ displaystyle \ omega _ {1} \ omega _ {2}}  ÂÂ$ menjadi vektor kecepatan sudut komposit dari titik yang berkaitan dengan ${\ displaystyle F_ {2}}  ÂÂ$ . Operasi ini bertepatan dengan penambahan biasa vektor, dan memberikan kecepatan sudut struktur aljabar dari vektor yang benar, bukan hanya pseudo-vektor.

Given a rotating frame of three unit coordinate vectors, all three must have the same angular velocity at all times. In such a framework, each vector can be considered a moving particle with constant scalar radius.

The rotating frame appears in the context of a rigid body, and a special tool has been developed for it: angular velocity can be described as a vector or equivalent as a tensor.

Consistent with the general definition, the frame angle velocity is defined as the angular velocity of one of three vectors (equal to all). The addition of angular velocity vectors to frames is also determined by the addition of ordinary vectors (linear motion composition), and may be useful for decomposing rotations as in dreadlocks. The components of a vector can be calculated as an instance of a parameter that defines a moving frame (Euler angle or rotation matrix). As in the general case, the addition is commutative: $Â\; Â{\displaystyle Â\; Â\; Â\; Â\; Â\; Â\; Â}$ _{Â Â Â Â Â Â Â Â Â Â ? Â Â Â Â Â Â Â Â Â Â Â Â Â ÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂ 1 Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â ? Â Â Â Â Â Â Â Â Â Â Â Â Â Â ÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂ 2 Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â = Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â ? Â Â Â Â Â Â Â Â Â Â Â Â Â Â ÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂ 2 Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â ? Â Â Â Â Â Â Â Â Â Â Â Â Â ÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂÂ 1 Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â {\ displaystyle \ omega _ {1} \ omega _ {2} = \ omega _ {2} \ omega _ {1}} Â Â} .

With Euler's rotation theorem, each rotating frame has a momentary rotation axis, which is the direction of the angular velocity velocity, and the angular velocity is consistent with the two-dimensional case.

Components of the vector of frames

Di sini ${\ displaystyle {\ mathbf {e}} _ {i}}  ÂÂ$ adalah kolom dari matriks frame, dan ${\ displaystyle {\ dot {\ mathbf {e}}} _ {i}}  ÂÂ$ turunan waktunya.

Komponen dari sudut Euler

The angular velocity pseudovector component is first calculated by Leonhard Euler using his Euler angle and the use of a frame between:

• One reference frame axis (precession axis)
• The node line of the moving frame with respect to the reference frame (nutation shaft)
• One moving frame axis (intrinsic rotation axis)

Euler proves that the pseudovector projection angular velocity on each of these three axes is a derivative of the corresponding angle (which is equivalent to decomposing an instantaneous rotation into three Euler rotations instantaneously). Therefore:

_{                                     u                                    Â 1                                                   Â               ?               ?      Â  ÂÂÂÂÂÂÂÂÂ,                                                               u                                     Â 2                                                   Â               ?               ?      Â  ÂÂÂÂÂÂÂÂÂ,                                                               u                                    Â 3                                {\ displaystyle {\ boldsymbol {\ omega}} = {\ dot {\ alpha}} {\ mathbf {u}} _ {1} {\ dot {\ beta}} {\ mathbf {u}} _ {2} {\ dot {\ gamma}} {\ mathbf {u}} _ {3}}  Â}

These basics are not orthonormal and difficult to use, but now velocity vectors can be changed to a fixed frame or to a moving frame only with a base change. For example, change to phone frame: ${\ displaystyle {\ boldsymbol {\ omega}} = ({\ dot {\ alpha}} \ sin \ beta \ sin \ gamma {\ dot { beta}} \ cos \ gamma) {\ mathbf {i}} ({\ dot {\ alpha}} \ sin \ beta \ cos \ gamma - {\ dot {\ beta}} \ sin \ gamma) {\ mathbf { j}} ({\ dot {\ alpha}} \ cos \ beta {\ dot {\ gamma}}) {\ mathbf {k}}} Â Â di mana                                                 saya                             ,                                  j                             ,                                  k                                      {\ displaystyle {\ mathbf {i}}, {\ mathbf {j}}, {\ mathbf {k}}}    adalah vektor satuan untuk bingkai yang ditetapkan dalam badan yang bergerak. Contoh ini telah dibuat menggunakan konvensi Z-X-Z untuk sudut Euler. Maps Angular velocity tensor kecepatan sudut Vektor kecepatan sudut                                    (                    ?                         x                             ,                    ?                         y                             ,                    ?                         z                             )                  {\ displaystyle {\ boldsymbol {\ omega}} = (\ omega _ {x}, \ omega _ {y}, \ omega _ {z})}    yang didefinisikan di atas dapat ekuivalen diekspresikan sebagai tensor kecepatan sudut , matriks (atau pemetaan linier) W = W ( t ) didefinisikan oleh:                         W          =                                  (                                                                 0                                                      -                                        ?                                             z                                                                                                                  ?                                             y                                                                                                                                                ?                                             z                                                                                              0                                                      -                                        ?                                             x                                                                                                                            -                                        ?                                             y                                                                                                                  ?                                             x                                                                                              0                                                          )                                      {\ displaystyle W = {\ begin {pmatrix} 0 & amp; - \ omega _ {z} & amp; \ omega _ {y} \\\ omega _ {z} & amp; 0 & amp; - \ omega _ {x} \\ - \ omega _ {y} & amp; \ omega _ {x} & amp; 0 \\\ akhir {pmatrix}}}    Ini adalah matriks rotasi yang sangat kecil. Pemetaan linear W bertindak sebagai                         (                    )                  {\ displaystyle ({\ boldsymbol {\ omega}} \ times)}    :                                                           Source of the article : Wikipedia Langganan: Posting Komentar (Atom) Most Visited Australian Army Cadets src: c8.alamy.com The Australian Army Cadets (AAC) is a youth organisation that is involved in training and adventurous activities in a mil... Calbayog src: i.ytimg.com Calbayog , officially Calbayog City , (Waray: Syudad san Calbayog ; Cebuano: ; Filipino: Calbayog City ) and is oft... MEMS magnetic field sensor src: slideplayer.com A MEMS magnetic field sensor is a small-scale microelectromechanical systems (MEMS) system for detecting and measur... Johannes Kepler src: media.npr.org Johannes Kepler ( ; German: [jo'han? s' k 27 December 1571 - November 15, 1630) was a German mathematician,... Sentosa src: static.asiawebdirect.com Sentosa , formerly called Pulau Blakang Mati , is a resort island in Singapore. It used to be a British mili... Blog Archives ▼  2018 (2254) Juli (146) Juni (267) Mei (373) April (265) Maret (150) Februari (421) Januari (632) ►  2017 (748) Desember (614) November (134) Diberdayakan oleh Blogger. Recent Post Formulir Kontak Nama Email * Pesan *   About Contact Privacy Policy Sitemaps Copyleft © 2017 Motion Info$